Circles
Video Courtesy of Kaplan GRE prep.
A circle is the set of all points in a plane that are the same distance from the center. A circle has 360°.
A segment from the center of the circle to the circle is called the radius r.
A segment that goes across a circle through the center is called the diameter d.
A diameter of a circle is twice the radius.
The circumference of a circle is the distance around the circle.
The formula for the circumference of a circle is C = 2πr = πd.

The formula for the area of a circle is A = πr2.
When using the formula for area, be sure the value you use is the radius, not the diameter. This is an occasional GRE trap.
When using an estimate for the value of π (pi), try π ≈ 3. If you need a more accurate estimate use:
π ≈ 3.14 ≈ \dfrac{22}{7}
Remember that the GRE is looking for reasoning, not long calculations. Leave π in your calculations. Only use an estimate for π when the answer choices contain numbers instead of π.
If you have any one of the radius, diameter, circumference or area of a circle, you can find all the other values.
Example
What is the radius of a circle if its circumference is numerically equal to twice its area?
Solution
Use the formulas to write an equation with circumference equal to 2(area), then solve for r.
C = 2πr A = πr2
2πr = 2(πr2), so r = 1.
Example
An automobile travels 2 miles. How many rotations are made by a tire with a 14-inch radius?
Solution
The number of rotations will be the distance divided by the circumference of the tire. Since there is division, keep the numbers in factored form rather than calculating completely.
The circumference of a tire with
r = 14 is 28π inches.
One mile = 5,280 feet = 12(5,280) inches. Two miles = 24(5,280) inches.
\dfrac{24 × 5,280}{28π} = \dfrac{24 × 5,280}{\dfrac{28 × 22}{7}} …Substitute 22/7 for π.
= \dfrac{24 × 5,280 × 7}{28 × 22} …Multiply by the reciprocal of the denominator.
= \dfrac{5,280 × 6 × 7}{7 × 22} = \dfrac{5,280 × 3}{11} …Factor and cancel.
The trick is that you can factor further because 5,280 is divisible by 11.
\dfrac{5,280 × 3}{11} = 480 × 3 = 1,140
The tire will rotate 1,140 times.
Example
A certain clock has a minute hand that is exactly 3 times as long as its hour hand. Point M is at the tip of the minute hand, and point H is at the tip of the hour hand. What is the ratio of the distance that point M travels to the distance that point H travels in 6 hours?
(A) 3 : 1 (B) 6 : 1
(C) 12 : 1 (D) 18 : 1
(E) 36 : 1
Solution
In 6 hours, point M on the minute hand travels 6 circumferences with radius = 3r.
So point M travels 6 × 2π3r = 36πr.
Point H on the hour hand only travels half way around the clock, half a circumference, with radius = r.
So point H travels (1/2) × 2πr = πr.
So the ratio is 36πr : πr, or 36 : 1.
The correct answer choice is (E).
Central Angles
A central angle has its vertex at the center of a circle, so it is formed by two radii.
An arc is a piece of a circle.
The measure of a central angle equals the measure of the intercepted arc.
In circle O, central angle ∠AOB = 60°. The measure of arc AB is 60°.
A sector is a “piece of a pie” created by a central angle and its arc. A sector has perimeter and area.

Example
The segments AC and BD are diameters. Find the measures of all the angles and arcs.

Solution
Using vertical angles, ∠2 = 130°.
AC is a line, so ∠1 + ∠2 = 180° and ∠1 = 50°.
Similarly, ∠3 = 50°.
Arcs have the same measures as their central angles, so
arc AD = arc BC = 50°.
Arc AB = arc CD = 130°.
Example
The area of the shaded region is 24π. Find the measure of arc ABC.
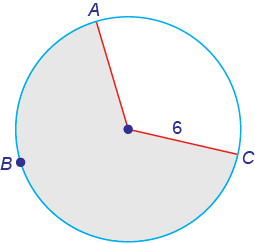
Solution
The ratio of the area of the sector to the area of the whole circle is the same as the ratio of the arc measures.
The area of the whole circle is A = πr2 = 36π. The area of the sector is 24π.
Set up a proportion.
\dfrac{sector}{circle} = \dfrac{24π}{36π} = \dfrac{\textit{x}}{360}
\\[3ex]\dfrac{2}{3} = \dfrac{\textit{x}}{360} so x = 240°. The measure of arc ABC = 240°.
Example
Find the perimeter and area of a 36° sector of a circle with radius 10.
Solution
A 36° sector is 36/360 = 1/10 of the circle.
The perimeter of the sector will be radius + radius
+ (1/10) circumference of circle.
The circumference of the circle is C = 2πr = 20π.
P = 10 + 10 + (1/10)20π = 20 + 2π
The area of the sector will be 1/10 the area of the circle.
The area of the circle is
A = πr2 = 100π.
A = (1/10)100π = 10π
Inscribed Angles
An inscribed angle has its vertex on the circle and is formed by two chords.
The measure of an inscribed angle equals the 1/2 the measure of the intercepted arc.
In circle O, ∠RTS = 50°. The measure of arc RS is 100°.
If two chords are congruent, then the arcs formed by the chords are congruent.
This is true if the chords are part of an inscribed angle, or just in the circle.
In circle O, TR = TU and arc TR = arc TU.

Example
In circle O above, ∠STU = 30°. What are the measures of arc SU, arc TR and arc TU?
Solution
Inscribed angle ∠STU = 30°, so arc SU = 60°.
The circle is 360°, so
arc RS + arc SU + arc TR + arc TU
= 360°.
Use x for the measure of
arc TR = arc TU.
100 + 60 + x + x = 360
2x = 200
x = 100 arc TR = arc TU = 100°
Example
What arc length is intercepted by an inscribed angle of 42° on a circle with r = 12 meters?
Solution
The measure of the 42° inscribed angle is 1/2 of the arc measure, so the arc is 84°.
The arc is a part of the whole circle, so the 84° is part of 360°. Find the fraction.
\dfrac{84}{360} = \dfrac{7 × 12}{3 × 10 × 12} = \dfrac{7}{30}\\[3ex]The circumference of the entire circle is 2πr = 24π.
So, the length of the arc is (\dfrac{7}{30})(24π).
Since it is a fraction, and has a 7 in the numerator, use π = 22/7. Remember to factor, not multiply, to avoid calculation.
(\dfrac{7}{30})(24π) ≈ (\dfrac{7}{30})(24)(\dfrac{22}{7})
= \dfrac{7 × 4 × 6 × 22}{5 × 6 × 7} = \dfrac{88}{5} = \dfrac{176}{10} = 17.6
The arc length is about 17.6 meters.
Example
Find the area of the shaded region.

Solution
The measure of the inscribed angle is 45°, so the measure of the arc is 90°.
The 90° tells you to look for a right triangle.
Drawing radius OC creates right triangle AOC. The shaded region is the sector minus the triangle.
Triangle AOC is an isosceles right triangle, so base = height = 4. The area is (1/2)(4)(4) = 8.
The sector has a central angle of 90°, so its area is 90/360 = 1/4 of the area of the circle.
The area of the sector is
(1/4)(π)(42) = 4π.
The area of the shaded region is 4π – 8.
If you need to calculate an estimate, factor first.
4π – 8 = 4(π – 2). Use 3.14 as an estimate for π.
4(π – 2) ≈ 4(3.14 – 2) = 4(1.14) = 4.56
Inscribed Polygons
An inscribed polygon is formed by chords so it has its vertices on the circle.
The GRE often uses inscribed polygons. You need to be able to combine the information about circles, angles and polygons.
There are two important relationships between angles of inscribed polygons.
- If one side of an inscribed triangle is a diameter, the triangle is a right triangle.

This is easy to see. The measure of an inscribed angle is equal to 1/2 of the intercepted arc. A diameter divides a circle into two 180° arcs. So the inscribed angle to the arc is 90°.
- Opposite angles of an inscribed quadrilateral add to 180°.

Also recall that the sum of the interior angles of a quadrilateral is 360°.
Example
A triangle with two sides 6 and 8 units long is inscribed in a circle. If the third side is a diameter, find the length of the diameter.
Solution
A triangle with one side the diameter is a right triangle.
The lengths of the sides this triangle are 6 : 8 : h. This is a multiple of a
3 : 4 : 5 right triangle, so the length of the hypotenuse that is a diameter is 10.
Example
Find the values of x, y and z.

Solution
The circle is the sum of the arcs, so start by finding 3z°.
360° = 110° + 130° + 54° + 3z° so 3z° = 66° and z = 22.
There are two methods to find the other two angles.
Method 1
The measures of inscribed angles are equal to half the sum of the opposite arcs.
Using the opposite arcs:
2x° = (1/2)(66° + 110°)
so 2x° = (1/2)176° and x = 44.
6y° = (1/2)(66° + 54°)
so 6y° = 60° and y = 10.
Method 2
Since the inscribed polygon is a quadrilateral, opposite angles of the quadrilateral add to 180°.
The measure of inscribed
∠C = (1/2)(130° + 54°) = 92°.
Add the opposite angles.
2x° + 92° = 180° so 2x° = 88° and x = 44.
The measure of inscribed
∠B = (1/2)(130° + 110°) = 120°.
6y° + 120° = 180°
so 6y° = 60° and y = 10.
Example
A square is inscribed in a circle of diameter 20. Determine the ratio of the area of the circle to the area of the square.
Solution
Sketch the figure.

You need to find the area of the circle and the square.
Be careful! Since diameter = 20,
r = 10, and the area of the circle is A = πr2 = 100π.
Notice a diameter divides the square into two 45° : 45° : 90° right triangles. The hypotenuse is the diameter so is 20. Each side of the square is
\dfrac{20}{\sqrt{2}} = \dfrac{20}{\sqrt{2}} \times \dfrac{\sqrt{2}}{\sqrt{2}} = 10\sqrt{2}
The area of the square is (10\sqrt{2})2
= 100 × 2 = 200.
The ratio of the areas is \dfrac{100\textit{π}}{200} = \dfrac{\textit{π}}{2}
Tangents to Circles
A tangent to a circle is a line that touches the circle in just one point.
A tangent is perpendicular to a diameter.

Angles formed by a chord and a tangent are 1/2 the measure of the arc.

Tangents from a common exterior point are congruent. The measure of the angle formed by the tangents is 1/2 the difference between the measures of the two arcs.

Example
In triangle ABC, find the measure of each arc of the inscribed circle.

Solution
Start with the right angle, ∠A. Let the measure of arc RST = x and the measure of arc RT = y.
x + y = 360 …The sum of the arcs is the full circle.
1/2(x – y) = 90 …The measure of the angle is 1/2 the difference of the arcs.
x – y = 180 …Simplify.
Solve the system of equations.
x + y = 360
x – y = 180
2x = 540 so x = 270.
x + y = 360 so y = 360 – 270 = 90. arc RT = 90°
The other angles ∠B and ∠C are both 45° angles, so arc RS = arc ST .
Let the measure of
arc RS = arc ST = z.
arc RS + arc ST + arc RT = 360°
z + z + 90 = 360
2z = 270 so z = 135.
arc RS = arc ST = 135°
Example (advanced)
In the circle in the Example above, the radius of the inscribed circle is 10. Find the area of triangle ABC.
Solution
Make a sketch.

To find the area, you need the length of any side or the length of segment AS as a height of triangle ABC.
Since a diameter is perpendicular to a tangent line, radii OS, OR and OT are perpendicular to the sides of triangle ABC.
The radii OR and OT form square AROT with side lengths of 10. The diagonal of the square forms 45° : 45° : 90° right triangles, so the length of the diagonal is
10\sqrt{2}.
So the length of segment AS is
10 + 10\sqrt{2}.
The segment AS is a height of triangle ABC, but the length of base BC is unknown.
Segment AS also forms two
45° : 45° : 90° right triangles in triangle ABC, triangle ASB and triangle ASC. So segments AS, SB and SC are equal.
So height AS = 10 + 10\sqrt{2} and base BC = 2(10 + 10\sqrt{2}).
The area of triangle ABC
= \dfrac{1}{2}[2(10 + 10\sqrt{2})](10 + 10\sqrt{2} )
= (10 + 10\sqrt{2})2
= [10(1 + \sqrt{2})]2 …Factor before multiplying.
= 102(1 + \sqrt{2})2 …Exponent rule (ab)2 = a2b2
= 100(1 + 2\sqrt{2} + 2)
= 100(3 + 2\sqrt{2})
If the answer options do not include \sqrt{2}, use 1.4 as an approximate value.
100(3 + 2\sqrt{2}) ≈ 100[3 + 2(1.4)]
= 100(5.8) = 580
Before attempting this problem, be sure to review this section on Quantitative Comparison questions.
https://www.youtube.com/watch?v=KDz5rnOBjRI&list=PLD0D070C218D8F5A3&index=24






