Vertical Angles
When two lines intersect, they create four angles.

The angles opposite one another are called vertical angles. Vertical angles are equal to each other.
Here 1 = 2, and 3 = 4. Another way to say this is that both “little angles” are equal to each other and both “big angles” are equal to each other.
Also, note that any pair of adjacent angles will always equal 180° (“little angle” added to any “big angle”).
Example
The figure shows two straight lines and a ray intersecting in the same point.
How many pairs of congruent angles less than 180° are there?

Solution
First look for all the vertical angles.
∠1 = ∠4 1 pair of congruent vertical angles
∠5 = ∠2 + ∠3 1 pair of congruent vertical angles
Then look for all the right angles.
∠2 is a right angle.
∠3 + ∠4 and ∠2 are supplementary angles. So ∠3 + ∠4 equals 180° – 90° = 90°.
1 pair of congruent right angles
So the total number of pairs of congruent angles is 1 + 1 + 1 = 3 pairs.
Parallel Lines
Two lines that never get closer to or farther away from each other and therefore never intersect are called parallel lines. The symbol for parallel lines is ||. In the figure to the right, AB || CD, which means line AB is parallel to line CD. Sometimes parallel lines are indicated by pointers on the lines.

When two parallel lines are cut by a third line, called a transversal, the three lines form a system of congruent angles.
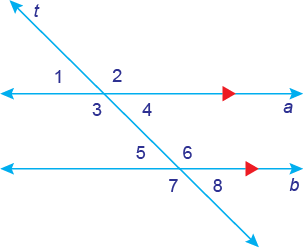
In this figure, vertical angles 1 = 4 and
5 = 8.
Because line a and line b are parallel,
1 = 5 and 4 = 8.
So the four angles are congruent:
1 = 4 = 5 = 8.
Similarly, the other four angles are also congruent:
2 = 3 = 6 = 7.
There are many terms to describe these congruent angles, such as “corresponding,” “alternate interior” or “alternate exterior,” but these terms are not used on the test. For the SAT, it is simply enough to know that all the “little angles” will be equal to each other and all the “big angles” will be equal to each other when parallel lines are cut by a transversal.
Also remember that any pair of adjacent angles will always equal 180° (“little angle” added to any “big angle”).
Angles that are on the same side of the transversal and “between” the parallel lines are supplementary and add to 180°. In the figure above, this means that ∠3 + ∠5 = 180° and ∠4 + ∠6 = 180°. This can be very useful when finding angles in polygons, such as rectangles, parallelograms and trapezoids.
Example
In the figure, ∠3 = 60°, AF || BE || CD, and AC || FE. Which angle measure(s) cannot be determined?

Solution
∠2 + ∠3 = 180°, so ∠2 = 120°.
Notice ∠1 with ∠2 and ∠3 with ∠4 are pairs of angles “between” parallel lines so each pair adds to 180°. Since you know the measures of ∠2 and ∠3, you can calculate
∠1 = 60° and ∠4 = 120°.
Also notice that ∠2 with ∠7 and ∠1 with ∠8 are also pairs of angles “between” parallel lines. Since you know the measures of ∠2 and ∠1, you can calculate
∠7 = 60° and ∠8 = 120°.
Since BE || CD, ∠5 with ∠6 are “between” parallel lines and ∠5 + ∠6 = 180°. But without more information, exact measures cannot be found.
Perpendicular Lines
When two lines intersect each other at a right angle (90°), the lines are perpendicular. The symbol for perpendicular is ⊥, and m ⊥ n means that line m and line n are perpendicular lines. Since perpendicular lines form right angles, there is often the right angle mark at the intersection.
Be careful not to assume that lines are parallel or perpendicular if it’s not stated explicitly. Don’t be fooled by appearance; you cannot assume that figures are drawn to scale. While the SAT isn’t likely to draw a figure grossly out of scale (like an acute angle that is actually obtuse), they do often draw lines that look parallel or perpendicular but aren’t (or vice versa).
Example
(a) If ∠4 = 85° and ∠6 = 95°, is line m parallel to line n?
(b) If ∠1 = ∠2 = ∠5, is line m parallel to line n? Are any of the lines perpendicular?
Note: Figure is not drawn to scale.
Solution
(a) Since
∠4 = 85° and ∠4 + ∠2 = 180°,
∠2 = 180° – 95° = 85°.
Since ∠2 = ∠6, line m is parallel to line n.
(b) Since ∠1 = ∠5, line m is parallel to line n.
Since ∠1 = ∠2, line m is perpendicular to line p.
Since line m is parallel to line n, line n is perpendicular to line p.
In this case, the figure is drawn to scale for (a) but not for (b), so it is important to rely only on the information given explicitly.







