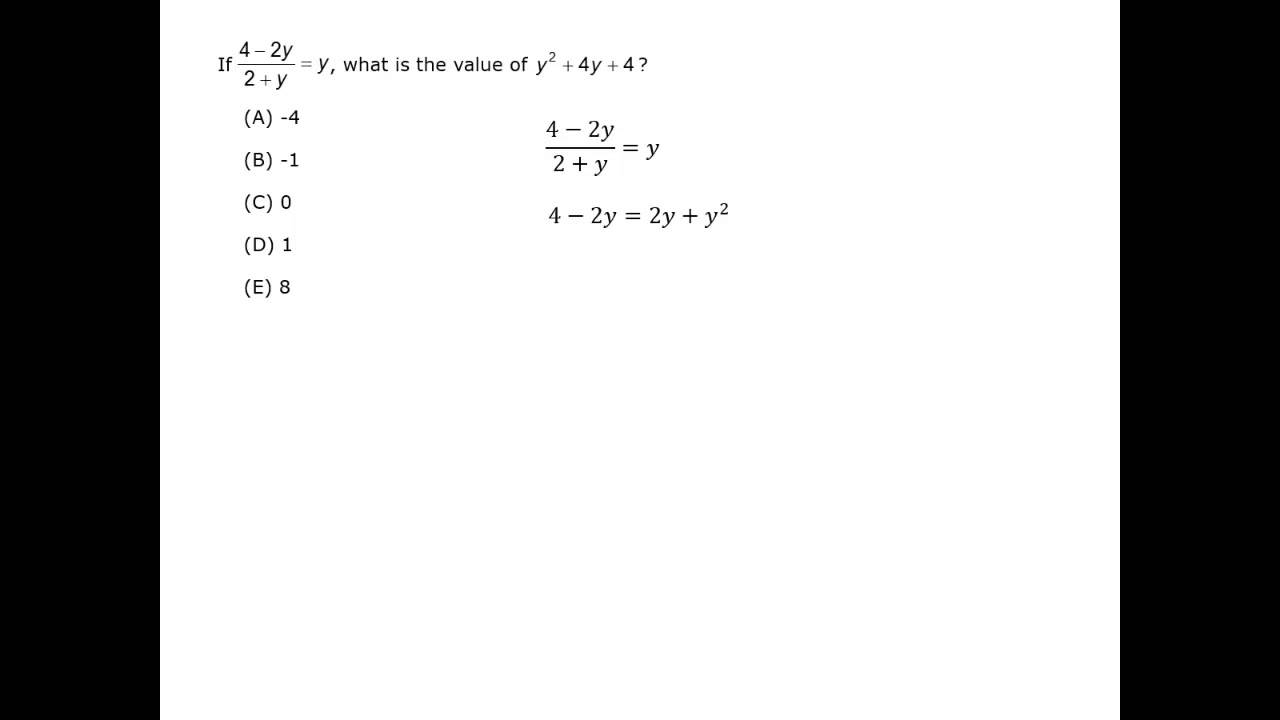Simplifying Expressions
You have already found the products of polynomials.
3y(y2 + 2y + 7) = 3y3 + 6y2 + 21y
(x + 3)(x + 2) = x2 + 5x + 6
You can use the same principles to divide polynomials.
dividing by a monomial
\dfrac{3\textit{y}^{\displaystyle{3}} + 6\textit{y}^{\displaystyle{2}} + 21\textit{y}}{3\textit{y}} = y2 + 2y + 7
dividing by a binomial
\dfrac{\textit{x}^{\displaystyle{2}} + 5\textit{x} + 6}{\textit{x} + 3} = x + 2
Two methods for dividing a polynomial by a monomial are factoring and dividing each term.
\dfrac{(6\textit{x}^{\displaystyle{3}} -\,8\textit{x}^{\displaystyle{2}} + 12\textit{x})}{2\textit{x}}
Simplify:
6x3 – 8x2 + 12x
Look for common factors.
6, 8, and 12 have the common factor 2. The common factor of x3, x2 and x is x.
6x3 – 8x2 + 12x = 2x(3x2 – 4x + 6)
\dfrac{(6\textit{x}^{\displaystyle{3}} \,-\, 8\textit{x}^{\displaystyle{2}} + 12\textit{x})}{2\textit{x}} = 3x2 – 4x + 6
\dfrac{25\textit{y}^{\displaystyle{4}} + 30\textit{y}^{\displaystyle{2}} \,-\, 20\textit{y}}{-5\textit{y}}
Simplify:
Divide each term.
\dfrac{25\textit{y}^{\displaystyle{4}} + 30\textit{y}^{\displaystyle{2}} \,-\, 20\textit{y}}{-5\textit{y}}
= \dfrac{25\textit{y}^{\displaystyle{4}}}{-5\textit{y}} + \dfrac{30\textit{y}^{\displaystyle{2}}}{-5\textit{y}} + \dfrac{-20\textit{y}}{-5\textit{y}}
= -5y3 – 6y + 4
Dividing By Binomials
Two methods for dividing a polynomial by a binomial are factoring and long division.
\dfrac{\textit{x}^{\displaystyle{2}} \,-\, 3\textit{x} \,-\, 4}{\textit{x} + 1}
Simplify:
Factor. \dfrac{\textit{x}^{\displaystyle{2}} \,-\, 3\textit{x} \,-\, 4}{\textit{x} + 1}
= \dfrac{(\textit{x} \,-\, 4)(\textit{x} + 1)}{(\textit{x} + 1)} = x – 4
\dfrac{\textit{x} \,-\, 7}{2\textit{x}^{\displaystyle{2}} \,-\, 11\textit{x} \,-\, 21}
Simplify:
Factor. \dfrac{\textit{x} \,-\, 7}{2\textit{x}^{\displaystyle{2}} \,-\, 11\textit{x} \,-\, 21}
= \dfrac{\textit{x} \,-\, 7}{(\textit{x} \,-\, 7)(2\textit{x} + 3)} = \dfrac{1}{2\textit{x} + 3}
\dfrac{(6\textit{y}^{\displaystyle{2}} + 7\textit{y} -\, 3)}{(3\textit{y} -\, 1)}
Simplify:
Use the format of long division:
—————2y + 3
3y – 1\overline{ \big)\,6y^{\displaystyle{2}} + 7y \,-\, 3}
xxxxxxxxx6y2 – 2y
xxxxxxxxxxx9y – 3
xxxxxxxxxxx9y – 3
xxxxxxxxxxxxxxx0
\dfrac{6\textit{y}^{\displaystyle{2}}}{3\textit{y}} = 2y
Multiply
3y – 1
by 2y and subtract.
7y – (-2y)
= 9y
\dfrac{9\textit{y}}{3\textit{y}} = 3
Multiply
3y – 1
by 3 and subtract.
\dfrac{(6\textit{y}^{\displaystyle{2}} + 7\textit{y} \,-\, 3)}{(3\textit{y} \,-\, 1)} = 2y + 3
Simplifying Exponential Expressions
Looking at the answers before starting to solve the problem is a good strategy for questions with exponents. You may match the answer format by just simplifying or estimating, rather than calculating to get a value.
Simplifying may be all that is needed. Answers will often have exponents rather than a numerical value.
An estimate needs to match the accuracy of the possible answer choices.
For any answer format, the first step is often to factor then simplify using the rules of exponents.
Remember to use Experiment as a technique if you’re not sure you remember a rule.
242 × 153 =
Solution
Answers will often contain the exponents rather than multiplying to get a value. Find the prime factors before multiplying the terms.
242 × 153
= (2 × 2 × 2 × 3)2 × (3 × 5)3
= (23 × 3)2 × (3 × 5)3
= (23)2 × 32 × 33 × 53
= 26 × 35 × 53
Evaluate: \dfrac{8^{\displaystyle{2}}}{4^{\displaystyle{5}}}
Solution
Find the prime factors to get the same base, then use the rule
\dfrac{\textit{a}^{\displaystyle{\textit{m}}}}{\textit{a}^{\displaystyle{\textit{n}}}} = am – n
\dfrac{8^{\displaystyle{2}}}{4^{\displaystyle{5}}} = \dfrac{{(2^{\displaystyle{3}})}^{{\displaystyle{2}}}}{{(2^{\displaystyle{2}})}^{{\displaystyle{5}}}} = \dfrac{2^{\displaystyle{6}}}{2^{\displaystyle{10}}}
= 26 – 10 = 2-4
To compare to the answer choices, you can evaluate further, to get a fraction or a decimal.
2-4 = \dfrac{1}{2^{\displaystyle{4}}} = \dfrac{1}{16} = 0.0625
\dfrac{2\textit{x}\textit{y}^{\displaystyle{3}}× 9\textit{y}}{{(3\textit{x}\textit{y})}^{\displaystyle{2}}}
Simplify:
Remember that on the GMAT, fractions might be on one line. So rewrite this as “built up”, then simplify.
Do the multiplication, then use the rule \dfrac{\textit{a}^{\displaystyle{\textit{m}}}}{\textit{a}^{\displaystyle{\textit{n}}}} = am – n for each base. (This is often called cancellation.)
\dfrac{{2\textit{xy}}^{\displaystyle{3}} × 9\textit{y}}{{(3\textit{xy})}^{\displaystyle{2}}} = \dfrac{18\textit{x}{\textit{y}^{\displaystyle{4}}}}{9{\textit{x}^{\displaystyle{2}}{\textit{y}^{\displaystyle{2}}}}} = \dfrac{2{\textit{y}^{\displaystyle{2}}}}{\textit{x}}Factor.
72 + 75
Solution
Factor by “undoing” using the distributive property.
72 + 75 = 72(1 + 73)
Factor.
32 + 122
Solution
Factor by “undoing” using the distributive property.
32 + 122 = 32 + (3 × 4)2
= 32 + 32 42 = 32(1 + 42)
If x = 52 + 53 + 54 + 55, what is the greatest prime factor of x?
Solution
Factor to find all the prime factors.
52 + 53 + 54 + 55
= 52(1 + 5 + 52 + 53)
= 52 (1 + 5 + 25 + 125)
= 52 (156)
= 52 (2 × 2 × 3 × 13)
So the greatest prime factor is 13.
A microorganism has a length of 5-7 meters. If 625 microorganisms of this length are arranged in a line, what is the length of the line in millimeters? (1m = 100 cm, 1 cm = 10 mm)
Solution
Factor 625.
625 = 252 = (52)2 = 54
Multiply. Use the rule
am × an = am + n
(5-7)(54) = 5-3 = \dfrac{1}{5^{\displaystyle{3}}} = \dfrac{1^{\displaystyle{3}}}{5^{\displaystyle{3}}}
= (\dfrac{1}{5}) × (\dfrac{1}{5}) × (\dfrac{1}{5})
= 0.2 × 0.2 × 0.2 = 0.008
Convert from meters to millimeters. 1 m = 100 cm, 1 cm = 10 mm, therefore
1 m = 1,000 mm (or 1 mm = 10-3 m), so multiply both sides by 1,000.
0.008 m = 8 mm
Complex Fractions
A complex fraction is a fraction that has a fraction in the numerator or denominator. In other words, it is a fraction divided by a fraction. Complex fractions can contain variable expressions. To simplify, multiply by the reciprocal, then factor and cancel any common factors.
\dfrac{\dfrac{\textit{x}^{\displaystyle{2}}}{9}}{\dfrac{3\textit{x}}{4}}
Simplify:
Use the reciprocal and multiply.
\dfrac{\dfrac{\textit{x}^{\displaystyle{2}}}{9}}{\dfrac{3\textit{x}}{4}} = \dfrac{\textit{x}^{\displaystyle{2}}}{9} \times \dfrac{4}{3\textit{x}} = \dfrac{4\textit{x}}{27}
\dfrac{\dfrac{9\textit{y}^{\displaystyle{2}}}{7}}{12\textit{y}}
Simplify:
Use the reciprocal and multiply.
\dfrac{\dfrac{9\textit{y}^{\displaystyle{2}}}{7}}{12\textit{y}} = \dfrac{9\textit{y}^{\displaystyle{2}}}{7} \times \dfrac{1}{12\textit{y}} …Division by 12y is multiplication by its reciprocal \dfrac{1}{12\textit{y}}.
\dfrac{3 \times 3 \times \textit{y} \times \textit{y}}{7} \times \dfrac{1}{3 \times 4 \times \textit{y}} = \dfrac{3\textit{y}}{28} …Factor, then cancel common factors.
\dfrac{\dfrac{\textit{x}^{\displaystyle{2}} + 7\textit{x}}{4}}{\dfrac{\textit{x}^{\displaystyle{2}} \,-\, 49}{\textit{x}}}
Simplify:
Use the reciprocal and multiply.
\dfrac{\dfrac{\textit{x}^{\displaystyle{2}} + 7\textit{x}}{4}}{\dfrac{\textit{x}^{\displaystyle{2}} \,-\, 49}{\textit{x}}}
= \dfrac{\textit{x}^{\displaystyle{2}} + 7\textit{x}}{4} \times \dfrac{\textit{x}}{\textit{x}^{\displaystyle{2}} \,-\, 49} …Multiply by the reciprocal.
\dfrac{\textit{x}(\textit{x} + 7)}{4} \times \dfrac{\textit{x}}{(\textit{x} + 7)(\textit{x} \,-\, 7)}
= \dfrac{\textit{x}^{\displaystyle{2}}}{4(\textit{x} \,-\, 7)} …Factor, then cancel common factors.
Adding Polynomial Fractions
When adding (or subtracting) algebraic fractions, follow the same process as adding number fractions. The first step is to write equivalent fractions that have the same (common) denominator.
- Find the least common denominator (LCD) of the fractions.
- Write equivalent fractions using the LCD.
- Add (or subtract) the numerators.
- Simplify and reduce the resulting fraction.
A quick method for finding the LCD is to multiply the denominators. But multiplying the denominators often gives a rather large expression. Instead of multiplying, factor the denominators. Use the least common multiple. The LCM will have one of each shared factor and all of the factors that are not shared. (To review LCD and LCM, see the sections on Divisibility and Fractions in the previous chapter.)
Find the least common multiple:
14s and 6s2
Solution
Factor.
14s = 2 × 7× s
6s2 = 2 × 3 × s × s

The LCM of 14s and 6s2 is
2 × 7× s × 3 × s = 42s2
Find the least common multiple:
y2– 25 and y2 + 6y + 5
Solution
Factor.
y2 – 25 = (y + 5)(y – 5)
y2 + 6y + 5 = (y + 1)(y + 5)
The LCM of y2 – 25 and
y2 + 6y + 5 is
(y + 5)(y – 5)(y + 1).
\dfrac{1}{2} + \dfrac{1}{2\textit{x}} + \dfrac{3}{\textit{x}^{\displaystyle{2}}} = 1
Solution
The least common denominator (LCD) is the least common multiple, 2x2.
\dfrac{1}{2} + \dfrac{1}{2\textit{x}} + \dfrac{3}{\textit{x}^{\displaystyle{2}}} = 1 …Multiply both sides by the LCD.
2x2\Bigg[\dfrac{1}{2} + \dfrac{1}{2\textit{x}} + \dfrac{3}{\textit{x}^{\displaystyle{2}}}\Bigg] = 2x2 …Use the distributive property.
\dfrac{2\textit{x}^{\displaystyle{2}}}{2} + \dfrac{6\textit{x}^{\displaystyle{2}}}{2\textit{x}} + \dfrac{10\textit{x}^{\displaystyle{2}}}{\textit{x}^{\displaystyle{2}}} = 2x2 …Cancel common factors in each fraction.
x2 + 3x + 10 = 2x2 …Subtract 2x2 from both sides and multiply by -1.
x2 – 3x – 10 = 0 …Factor.
(x – 5)(x + 2) = 0
so x = 5 and x = -2
\dfrac{5\textit{y}}{\textit{y} \,-\, 2} = \dfrac{15}{\textit{y}} + \dfrac{10}{\textit{y} \,-\, 2}
Solution
The least common denominator is y × (y – 2) = y(y – 2).
y(y – 2) \Bigg[\dfrac{5\textit{y}}{\textit{y} \,-\, 2}\Bigg]
= y(y – 2) \Bigg[\dfrac{15}{\textit{y}} + \dfrac{10}{\textit{y} \,-\, 2}\Bigg] …Multiply both sides by the LCD. Cancel common factors.
5y2 = 15(y – 2) + 10y …Use the distributive property.
5y2 = 15y – 30 + 10y …Set equations equal to zero.
5y2 – 25y + 30 = 0 …Divide by 5.
y2 – 5y + 6 = 0 …Factor.
(y – 2)(y – 3) = 0 so y = 2 and y = 3.
Check the answers by substituting in the original equation. If y = 2, the denominator y – 2 is equal to zero. Division by zero is undefined. So the only solution is y = 3.
How Many Solutions?
The number of solutions for polynomial equations can vary. Some equations have infinite solutions or no solutions. Therefore, you need to be careful when performing operations on an equation that you don’t lose a possible solution or get an extraneous solution, which is a solution that does not satisfy the original equation. The value y = 2 in the Example above is an extraneous solution.
You might lose a solution if you divide both sides by the variable, and you might get an extraneous solution if you square both sides of an equation.
\sqrt{5\textit{x}} + 5 = 0
Solution
\sqrt{5\textit{x}} + 5 = 0 …Subtract 5 from both sides.
\sqrt{5\textit{x}} = -5 …It looks like the next step is to square both sides, so x = 5
But on the GMAT, a square root is never a negative number. There are no solutions.
4x3 = x
Solution
4x3 = x …It looks like the way to solve the equation is to divide both sides by x.
4x3 = x 4x2 = 1 x2 = \dfrac{1}{4}
x = \dfrac{1}{2} and x = \dfrac{-1}{2}
But by dividing both sides by x, you lose a value for x.
4x3 = x 4x3 – x = 0
x(4x2 – 1) = 0 x(2x + 1)(2x – 1) = 0
So x = 0 is another solution. The solutions are x = 0, x = \dfrac{1}{2} and
x = \dfrac{-1}{2}.
16 – y2 = 10(4 + y)
Solution
16 – y2 = 10(4 + y) …Factor.
(4 + y)(4 – y) = 10(4 + y) …It looks like the next step is to divide both sides by 4 + y.
4 – y = 10 y = 4 – 10 = -6
But by dividing both sides by 4 + y, you lose a value for y.
16 – y2 = 10(4 + y)
16 – y2 = 40 + 10y
y2 + 10y + 24 = 0
(y + 6)(y + 4) = 0
y = -6 and y = -4
So y = -4 is another solution.
\sqrt{2\textit{x}} = x – 4
Solution
\sqrt{2\textit{x}} = x – 4 …Square both sides.
2x = (x – 4)2 = x2 – 8x + 16
x2 – 10x + 16 = 0
(x – 2)(x – 8) = 0
x = 2 and x = 8
Check the answers by substituting in the original equation.
If x = 2, then \sqrt{2\textit{x}} = x – 4 becomes \sqrt{4} = 2 – 4 = -2 . So x = 2 is an extraneous solution.
The only solution is x = 8.
If \dfrac{\textit{x}}{\textit{y}} = 16 and \dfrac{\textit{x}}{\textit{y}^{\displaystyle{2}}} = 8, what is xy?
Solution
\dfrac{\textit{x}}{\textit{y}} = 16 …Multiply both sides by y.
x = 16y
\dfrac{\textit{x}}{\textit{y}^{\displaystyle{2}}} = 8 …Multiply both sides by y2.
x = 8y2
16y = 8y2 …Set the two values of x equal.
2y = y2
y2 – 2y = 0 y(y – 2) = 0
y = 2 and y = 0
You are solving for xy. Substitute the values of y in either equation to find x.
\dfrac{\textit{x}}{\textit{y}} = 16 and y = 2
\dfrac{\textit{x}}{2} = 16 x = 32
\dfrac{\textit{x}}{\textit{y}} = 16 and y = 0
\dfrac{\textit{x}}{0} = 16 …Division by zero is undefined, so y = 0 is an extraneous solution.
The only solution is
xy = 32 × 2 = 64.
\dfrac{\textit{x}}{\textit{y}} = 16 Multiply both sides by y. x = 16y
\dfrac{\textit{x}}{\textit{y}^{\displaystyle{2}}} = 8 Multiply both sides by y2. x = 8y2
16y = 8y2 Set the two values of x equal.
2y = y2 y2 – 2y = 0 y(y – 2) = 0 y = 2 and y = 0
You are solving for xy. Substitute the values of y in either equation to find x.
\dfrac{\textit{x}}{\textit{y}} = 16 and y = 2 \dfrac{\textit{x}}{2} = 16 x = 32
\dfrac{\textit{x}}{\textit{y}} = 16 and y = 0 \dfrac{\textit{x}}{0} = 16 Division by zero is undefined, so y = 0 is an extraneous solution.
The only solution is xy = 32 × 2 = 64.
Polynomials and Radicals
You can apply the properties of multiplying and dividing polynomials to simplify equations with radicals. Remember that the simplest form of an expression does not have radicals in the denominator. (To review rules of radicals, see the section on Roots in the previous chapter.)
\dfrac{6}{2\sqrt{3}}
Simplify:
To simplify, multiply by a fraction equal to 1 with numerator and denominator equal to the radical.
\dfrac{6}{2\sqrt{3}} = \dfrac{6}{2\sqrt{3}} \times \dfrac{\sqrt{3}}{\sqrt{3}} = \dfrac{6\sqrt{3}}{2 \times 3} = \sqrt{3}
\sqrt{\dfrac{\textit{x}^{\displaystyle{2}}}{2}}
Solution
To simplify, multiply by a fraction equal to 1 with numerator and denominator equal to the radical.
\sqrt{\dfrac{\textit{x}^{\displaystyle{2}}}{2}} = \dfrac{\sqrt{\textit{x}^{\displaystyle{2}}}}{\sqrt{2}} …First apply the rule of exponents (\dfrac{\textit{a}}{\textit{b}})n = \dfrac{\textit{a}^{\displaystyle{\textit{n}}}}{\textit{b}^{\displaystyle{\textit{n}}}}
\\[3ex]\dfrac{\sqrt{\textit{x}^{\displaystyle{2}}}}{\sqrt{2}} = \dfrac{|\textit{x}|}{\sqrt{2}} \times \dfrac{\sqrt{2}}{\sqrt{2}} = \dfrac{|\textit{x}| \times \sqrt{2}}{2}
\big(\sqrt{\textit{x}} \,-\, \sqrt{\textit{y}}\big) \big(\sqrt{\textit{x}} + \sqrt{\textit{y}}\big)
Solution
\big(\sqrt{\textit{x}} \,-\, \sqrt{\textit{y}}\big) \big(\sqrt{\textit{x}} + \sqrt{\textit{y}}\big)= x – y …Use
(a + b)(a – b) = a2 – b2
Variables as Exponents
Apply the properties of polynomials and exponents when exponents are variables. Rewrite terms to have the same base. Write an equation from the exponents. (To review, see the section on Exponents in the previous chapter.)
52y × 5y – 1 = 25
Solution
52y × 5y – 1 = 25 …Simplify each side of the equation.
52y × 5y – 1 = 52y + y – 1 = 53y – 1 …On the left side of the equation, use am × an = am + n.
25 = 52 …On the right side of the equation, factor 25 so both bases are 5.
53y – 1 = 52 …Use the simplified expressions to write the original equation.
3y – 1 = 2 …Write just the exponents in an equation and solve.
y = 1
\dfrac{2}{4^{\displaystyle{\textit{x}}}} = 32
Solution
\dfrac{2}{4^{\displaystyle{\textit{x}}}} = 32
\dfrac{2}{4^{\displaystyle{\textit{x}}}} = \dfrac{2}{(2^{\displaystyle{2}})^{\displaystyle{\textit{x}}}} = \dfrac{2}{2^{\displaystyle{2\textit{x}}}} = 2^{\displaystyle{1 \,-\, 2\textit{x}}} …Use (am)n = amn.
32 = 25 …On the right side of the equation, factor 32 so both bases are 2.
21 – 2x = 25 …Substitute the simplified expressions in the original equation.
1 – 2x = 5 …Write just the exponents in an equation and solve.
x = -2
Factor: xa + xa + 1
Solution
To simplify, find the common factor. If the common factor isn’t clear, use the Plugging In method to try some numbers.
Let a = 2. Then xa + xa + 1 becomes x2 + x3. The common factor is x2, so x2 + x3 = x2(1 + x).
xa + xa + 1 = xa(1 + x)
The common factor is xa.
Video Quiz
Complex Expressions
Best viewed in landscape mode
3 questions with video explanations
100 seconds per question
Are you sure you want to refresh the question?
https://www.youtube.com/watch?v=bjwSSIP3ceo
https://www.youtube.com/watch?v=OWJ7ZlTZsWo
https://www.youtube.com/watch?v=ZLVVd3C6umk
https://www.youtube.com/watch?v=6CnmVwI2Szk&list=PL6F58D00ADB3C0A85&index=2
Before attempting these problems, be sure to review this section on data sufficiency questions.
https://www.youtube.com/watch?v=QHFiS1Z3XGU
https://www.youtube.com/watch?v=4yF4-EZtcMc
https://www.youtube.com/watch?v=Y16-ydvWyFo
https://www.youtube.com/watch?v=k17Ddnq1hOo